単軸引張り試験データを使った超弾性材料の設定方法
Altair Employee <?xml version="1.0" encoding="UTF-8"?>
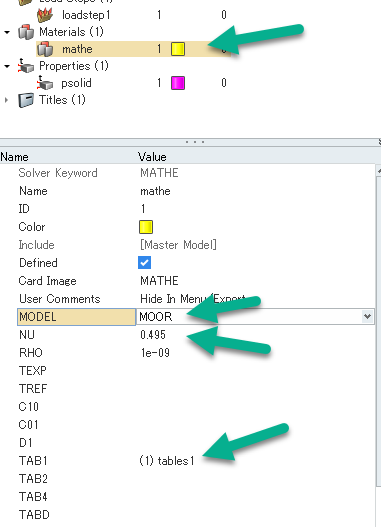
TAB1 は TABLES1 です。HyperWorks X で見るとこんな感じです。
.out ファイルには、同定されたパラメータが出てきます（ Mooney-Rivlin の例）
Hyperelastic Material Constants
Constants (fitted by using user provided test data) related to the DEVIATORIC strain energy function:
C10 C01
0.35430520 -0.19456034
テストデータと、材料モデル式との比較も出てきます。
Uniaxial test data available
Strain Stress(test) Stress(OptiStruct)
0.10000000E-01 0.10000000E-01 0.96045470E-02
0.20000000E-01 0.20000000E-01 0.19244842E-01
0.30000000E-01 0.30000000E-01 0.28915313E-01
0.40000000E-01 0.40000000E-01 0.38610857E-01
0.50000000E-01 0.50000000E-01 0.48326794E-01
0.60000000E-01 0.60000000E-01 0.58058839E-01
0.70000000E-01 0.70000000E-01 0.67803068E-01
0.80000000E-01 0.80000000E-01 0.77555891E-01
0.90000000E-01 0.90000000E-01 0.87314023E-01
0.11000000E+00 0.10000000E+00 0.10683446E+00
0.12000000E+00 0.11000000E+00 0.11659151E+00
0.13000000E+00 0.12000000E+00 0.12634334E+00
0.14000000E+00 0.13000000E+00 0.13608786E+00
0.15000000E+00 0.14000000E+00 0.14582318E+00
0.16000000E+00 0.15000000E+00 0.15554758E+00
0.17000000E+00 0.16000000E+00 0.16525949E+00
0.18000000E+00 0.17000000E+00 0.17495750E+00
0.19000000E+00 0.18000000E+00 0.18464033E+00
0.20000000E+00 0.20000000E+00 0.19430683E+00
0.10000000E+01 0.10000000E+01 0.89958762E+00
どの程度のひずみまで安全に計算できるか、Drucker の安定性についての調査も出てきます。あまりよろしくないですね。
Hyperelastic Material Drucker Stability Check Results (MATHE # 1):
Uni-axial tension: Becoming unstable at the nominal strains 0.35000000
Uni-axial compression: Becoming unstable at the nominal strains 0.26580000
Bi-axial tension: Becoming unstable at the nominal strains 0.16700000
Bi-axial compression: Becoming unstable at the nominal strains 0.13920000
Planar tension: Becoming unstable at the nominal strains 0.30200000
Planar compression: Becoming unstable at the nominal strains 0.23150000
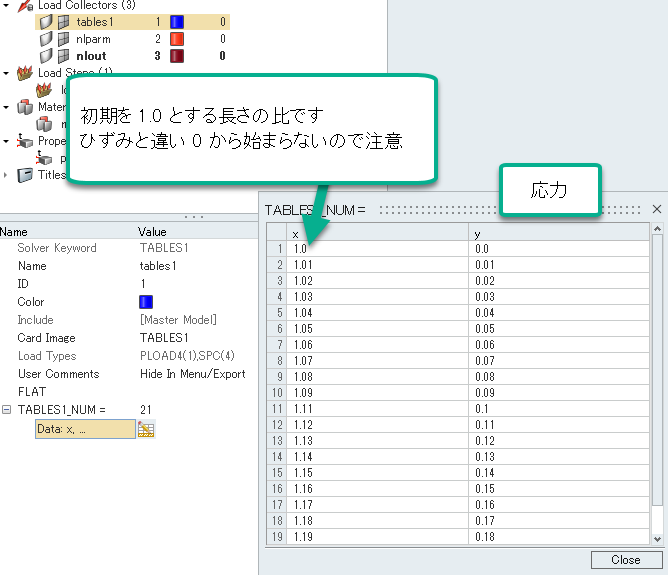
設定例はこちら（カーブはでたらめです）: